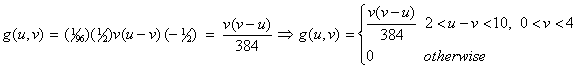11. Incorrect. The answer is false not true. It is possible to obtain such a formula by making a change of variable: u = x + 2y and v = x. The simultaneous solution is x = v and y = 1/2(u - v). Thus, after changing variables, the integration region changes from 0 < x < 4 and 1 < y < 5 (region E), changes to 0 < v < 4 and 2 < u - v < 10 (region D), which is shown shaded in the following figure:

We have to apply Theorem 2-4, p.45 in Schaum's Outlines Probability and Statistics. Let x and y be continuous random variables having joint density function f(x,y,). Let us define u = φ₁(x, y), v = φ₂(x, y) where x = ψ₁(u, v), y = ψ₂(u, v). The joint density function of u and v is given by g(u, v) where:

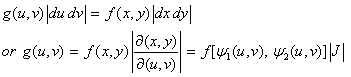
where J = Jacobian determinant (or simply Jacobian) that is given by:

.

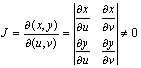The determinant of 2x2 matrix

is equal to: ad - cb. In the problem, the Jacobian is:

So, the change of variable allows us to obtain: